Hausdorff Measure, Dimension, and Analytic Capacity
At the mid of Spring semester 2024, I began doing some readings with Prof. Henri Martikainen on Hausdorff measures and dimensions, fractals, and analytic capacity using Xavier Tolsa’s Analytic Capacity, the Cauchy Transform, and Non-homogeneous Calderón–Zygmund Theory, Pertti Mattila’s Geometry of sets and measures in Euclidean spaces: Fractals and rectifiability, and K. J. Falconer’s The Geometry of Fractal Sets. I also consulted Prof. Steven Krantz on more details of chapters in The Theory and Practice of Conformal Geometry.
Note on measures, dimensions, and AC.
Two simple python codes generate the following plots for Mandelbrot and Julia sets.
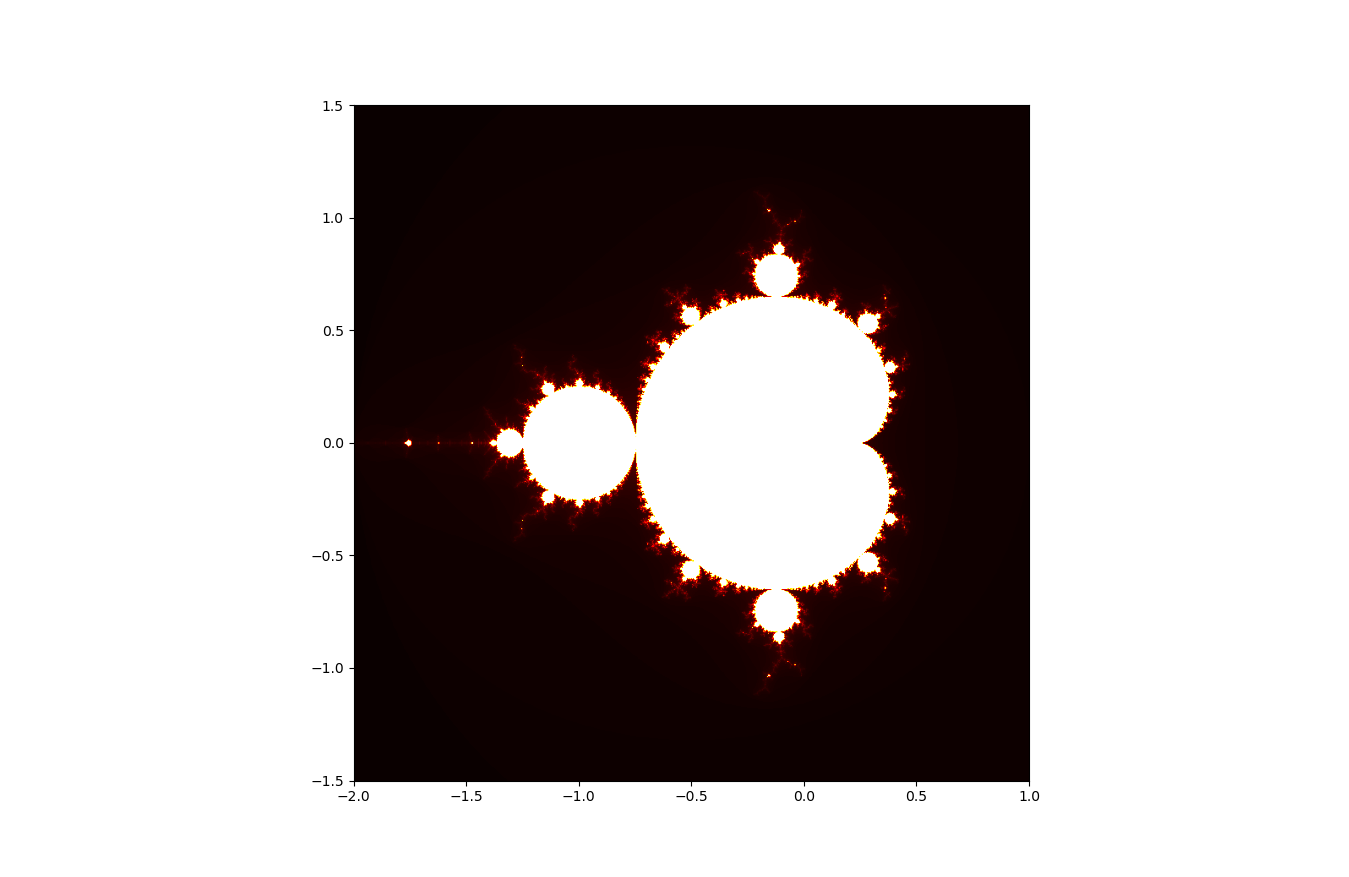
Mandelbrot set
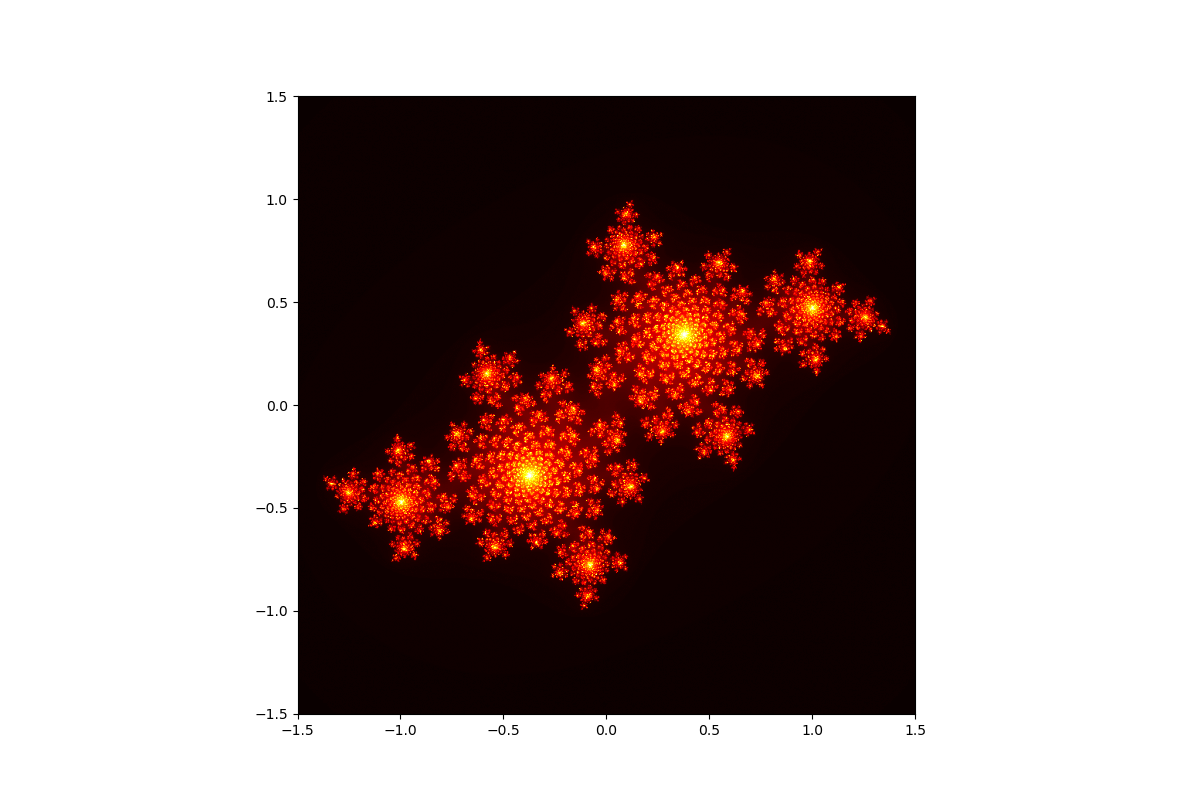
Mandelbrot set